Euclidean Relativity  (a.k.a. 'proper time physics' or 'proper time geometry')

Relativity theory traditionally uses the Minkowski hyperbolic framework. Euclidean relativity proposes a circular geometry as alternative that uses proper time   as the fourth spatial dimension. Other common elements in Euclidean relativity are the Euclidean (++++) metric as opposed to the traditional Minkowski (+---) or (-+++) metric, and the universal velocity c for all objects in 4D space-time.

The Euclidean metric is derived from the Minkowski metric by rewriting

    

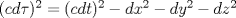
into the equivalent

     .

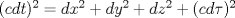The roles of time t and proper time  have switched so that proper time  becomes the coordinate for the 4th spatial dimension. The universal velocity c appears from the regular time derivative

     .

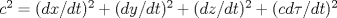The switch affects all relativistic formulas for displacement, velocity, acceleration and so on in a similar way; invariants are based on t while vector components representing the 4th dimension are based on  . Many Euclidean interpretations introduce time t as a parameter for tracking velocity and change, while in some articles it is treated as a fifth dimension.

The approach differs from "Wick rotation" or complex Euclidean relativity. Wick rotation replaces time t by it, which also yields a positive definite metric but it maintains proper time  as the invariant value whereas in Euclidean relativity  becomes a coordinate.

The Euclidean geometry is consistent with Minkowski based relativity. The hyperbolic Minkowski geometry turns into a rotation in 4D circular geometry where length contraction and time dilation result from the geometric projection of 4D properties to our 3D space.

The justification for the Euclidean approach is twofold: it makes relativity accessible in an intuitive way and it opens new opportunities to further develop relativity theory. 

Below are a number of references to articles on Euclidean relativity published by various authors. Each author approaches the topic in his own way and individual interpretations were often developed independently without knowledge of the other authors. First fundamental work came from the Dutch mathematician Hans Montanus in the beginning of the 90's. 

References:

Prof. Robert d'E Atkinson  Probably the first exploration of Euclidean relativity in history. General Relativity in Euclidean Terms (Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences, Volume 272, Issue 1348, pp. 60-78, 02-1963(!)). Does not yet use proper time  as fourth spatial dimension because it only deals with general relativity with stationary mass particles. Dr. R. G. Newburgh, Dr. T. E. Phipps  US Air Force research paper. A Space-Proper Time Formulation of Relativistic Geometry (Air Force Cambridge Research Laboratories, Office of Aerospace Research, U.S. Air Force, 1969) Seems like the first proposal to use proper time  as fourth spatial dimension. See also this document for a complete listing and biography of Dr. Phipps. Hans Montanus PhD  Introduces the difference between Relative and Absolute Euclidean space-times (REST versus AEST), while he favors the latter. Special relativity in an absolute Euclidean Space-Time (Physics Essays, vol 4, nr 3, 1991) The Fizeau experiment in an absolute Euclidean Space-time (Physics Essays, vol 5, nr 4, 1992) A new concept of time (Physics Essays, vol 6 nr 4, 1993) General relativity in an absolute Euclidean space-time (Physics Essays, vol 8, nr 4, 1995) Electrodynamics in an absolute Euclidean space-time (Physics Essays, vol 10, nr 1, 1997) Arguments against the general theory of relativity and for a flat alternative (Physics Essays, vol 10 nr 4, 1997) Compton scattering, pair annihilation, and Pion decay in an absolute Euclidean space-time (Physics Essays, vol 11, nr 2, 1998) A Geometrical Explanation for the Deflection of Light (Physics Essays, vol 11, nr 3, 1998) Hyperbolic Orbits in an Absolute Euclidean Space-time (Physics Essays, vol 11 nr 4, 1998) Proper Time Physics (PDF) (Hadronic J.22:625-673,1999) Galactic Rotation and Dark Matter in an Absolute Euclidean Space-time (Physics Essays, vol 12 nr 2, 1999) Proper-Time Formulation of Relativistic Dynamics (Found. Phys. 31, Issue 9, Sep 2001, Pages 1357 - 1400) Flat Space Gravitation (Found. Phys. 35, Issue 9, Sep 2005, Pages 1543 - 1562) Talk at the IARD conference 2004. Prof. Jose Almeida  An Euclidean extrapolation to general relativity, explaining geodesic motion of objects as a result of a 4D refractive index, hence the alternative name '4D Optics'. Almeida considers 4D space-time as a Euclidean null-subspace of a 5D space-time with metric (-++++). The approach allows a treatment of  mass particles in 4D equivalent to photons in 3D, which is supplemented by considering particle worldlines as normals to wavefronts. An alternative to Minkowski space-time (arXiv:gr-qc/0104029, 2001) 4-Dimensional optics, an alternative to relativity (arXiv:gr-qc/0107083, 2001); A theory of mass and gravity in 4-dimensional optics (arXiv:physics/0109027, 2001) K-calculus in 4-dimensional optics (arXiv:physics/0201002, 2002) Prospects for unification under 4-dimensional optics (arXiv:hep-th/0201264, 2002) Unification of classic and quantum mechanics (arXiv:physics/0211056 ,2002) Maxwell's equations in 4-dimensional Euclidean space (arXiv:physics/0403058, 2004) Euclidean formulation of general relativity (arXiv:physics/0406026, 2004) The null subspace of G(4,1) as source of the main physical theories (arXiv:physics/0410035, 2004) Talk at the Moscow conference Number Time Relativity 2004 Talk at the PIRT IX conference Londen 2004. Choice of the best geometry to explain physics (arXiv:physics/0510179, 2005) Monogenic functions in 5-dimensional spacetime used as first principle: gravitational dynamics, electromagnetism and quantum mechanics (arXiv:physics/0601078, 2006) How much in the Universe can be explained by geometry? (arXiv:0801.4089, 2008) Prof. Alexander Gersten  Uses the term 'Mixed Space' to refer to the space where time t and proper time have changed place. Probably the first one to recognize the value of Montanus' work. Talk at the IARD conference 2002. Euclidean Special Relativity (Found. Phys. 33, 2003, Pages 1237-1251) Dr. Richard Gauthier  Also proposes a modification in the Minkoswki spacetime metric where time t and proper time change place. The idea originated from a study of the relativistic energy-momentum components of a theoretical model for the electron being composed of a circulating spin-1/2 charged photon. Electrons are spin 1/2 charged photons generating the de Broglie wavelength (Proceedings of SPIE Vol. 9570 95700D-1, 2015) Derivation of the Inertial Mass m = E o / c 2 of an Electron Composed of a Circling Spin-1/2 Charged Photon (web, 2017) Inertia explained (web, 2017) Relativity Simplified (web, 2017) Carl Brannen MSc. Emphasis on the geometry and mathematics (geometric or Clifford algebra) that could be used as a basis for Euclidean relativity. The Proper Time Geometry (pdf, ver 1.0 10/19/2004) Phase Velocity of de Broglie Waves (pdf, ver 1.0 11/20/2004) The Geometry of Fermions (pdf, ver 1.01, 10/21/2004) The Geometric Speed of Light (pdf, ver 1.0 11/07/2004) Nonlinear Waves on the Geometric Algebra (pdf, ver 1.1 12/02/2004) Homepage of Carl with various other papers on particle physics. Dr. Giorgio Fontana  Summarizes the results of Montanus, Gersten and Almeida in his first article and extends this with some more speculative thoughts The Four Space-times Model of Reality (arXiv.org, physics/0410054A) Hyperspace for Space Travel, Video of presentation at the STAIF 2007 by Dr. Eric Davis (American Institute of Physics, C.P. 880, pp. 1117-1124) Gravitational Waves in Euclidean Space (PDF) (Excerpt from AIP Conference Proceedings 969, 1055 (2008)) On the foundations of Gravitation Inertia and Gravitational Waves (Scribd) Extending Maxwell's equations to Euclidean relativity in 5D Towards an Unified Engineering Model for Long (and short?) Range Forces and Wave Propagation (Powerpoint presentation) Dr. Anthony Crabbe  As an alternative to the traditional Minkowski hyperbolic geometry the author uses 'Circular Function Geometry' (CFG), which is natural for many Euclidean interpretations of special relativity. Alternative conventions and geometry for Special Relativity (Annales de la Fondation Louis de Broglie Vol 29 no 4, 2004) The Limitations of the Minkowski Model of Space-time talk at the 13th Triennial Conference of the International Society for the Study of Time (Monterery, CA July 28-Aug 3 2007) Albert Zur Ph.D. A comprehensive study of four-vectors based on the characteristic properties of Euclidean relativity in momentum-space. A Theory of Special Relativity Based on Four-Displacement of Particles Instead of Minkowski Four-Position  (PDF, viXra:1807.0237) Rob F.J. Van Linden BSc.Rob van Linden, van Linden RFJ Minkowski versus Euclidean 4-vectors (web, feb 2006) Associating 4-vectors with geometric properties in Euclidean space-time. Dimensions in Special Relativity. An Euclidean Interpretation (Galilean Electrodynamics Vol 18 Nr 1, jan/feb 2007) Essentials of the approach in Euclidean relativity. Propulsion without propellant using four-momentum of photons in Euclidean special relativity (web, apr 2008) An alternative method to accelerate particles or objects is described. It uses principles of 4D momentum that follow from Euclidean special relativity. Relativity Simplified Simplified and popularized description of the essentials of Euclidean relativity. The Universe as a Multi-Dimensional Fractal Speculative description of a fractal-like universe, based on the geometry of Euclidean relativity. It suggests a hierarchical ordering of the four forces of Nature together with their fermions and bosons through their number of dimensions and provides answers to the expansion of the universe and its missing mass. Dr. Phillips V. Bradford  Characteristic elements of Euclidean relativity, using proper time and universal velocity c for all objects in space-time. Alternative ways of looking at physics, with amongst others A space-time, geometric interpretation of the beta factor in Special Relativity. Richard D. Stafford Ph.D., Resolution of the Relativity/Quantum Mechanics Conflict (on Web Archive) Uses Euclidean space-time with   as fourth dimension to solve a common problem with the perception of reality. Radovan Machotka Ph.D., Euclidean Model of Space and Time (Journal of Modern Physics 9, 1215-1249, may 2018) Shows that four-dimensional Euclidean space allows explanation of known relativistic effects that are now explained in pseudo-Euclidean spacetime by Einstein's Special Theory of Relativity. Vu B Ho Advanced Study, 9 Adela Court, Mulgrave, Victoria 3170, Australia, Euclidean Relativity (web) Presents in more details the formulation of Euclidean relativity. Prof. Dr. Markolf H. Niemz Physics at a Crossroads: Weird Concepts or Orthogonal Projections (Preprints 2022, 2022070399). Discloses an issue in special and general relativity (“the subjective concepts applied in SR and GR fail to solve fundamental mysteries of physics”) and formulates the physics of Euclidean relativity. Niemz suggests that we replace subjective concepts (spatial, temporal, wave, particle, force, field) with objective concepts (pure distance, pure energy, process). He shows that only Euclidean relativity provides a “holistic view” (view from all possible perspectives at the same instant in time). Niemz solves 15 fundamental mysteries (including time’s arrow and the Hubble constant tension). He also declares four concepts of today’s physics obsolete (including dark energy and non-locality). Dr. Takuya Yamashita Theoretical evidence for principles of special relativity based on isotropic and uniform four-dimensional space (Preprints 2023, 2023051785). The author explains relativistic phenomena and the concept of time by introducing a 4-dimensional real space with regular time t as parameter to derive velocities and displacements therein. The fourth spatial dimension corresponds to proper time   in classical special relativity, as is common in similar articles on Euclidean relativity. Wenhui Zheng B.Sc. A Euclidean geometric model that has a maximum speed c (Physics Essays Vol. 36, Nr 1, March 2023, pp. 117-122). Another proposal for a standard Euclidean geometric model that explains the constant speed c and the geometric mechanism that causes time dilation and length contraction effects. It uses a new coordinate transformation and velocity addition formula that is consistent with the Michelson–Morley experiment and the linear Sagnac experiment. Note on articles by Dr. Frans-Guenter Winkler (ResearchGate, arXiv): although the same terms Euclidean special and general relativity are used, the geometry of the model is different. It maintains t as fourth dimension and   as invariant, yet uses a (++++) metric. The approach falls outside the scope of this page.

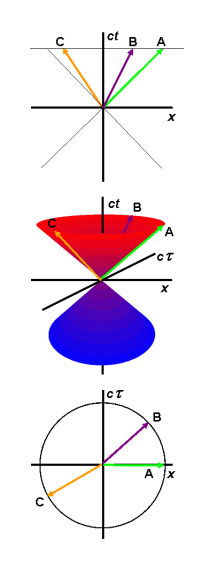
Hans Montanus' visualization of the relation between Minkowski and Euclidean diagrams (From: Proper Time Physics, Hadronic Journal 22, 625-673, 1999)

Books: 

Lewis Carroll Epstein Relativity Visualized (Insight Press 1981) A popular explanation of special relativity using concepts of Euclidean relativity. Brian Greene The Fabric of the Cosmos: Space, Time, and the Texture of Reality (Knopf New York 2004) Typical elements of Euclidean Relativity are used at various places in the book to explain relativistic effects. Thomas W. Sills What Einstein did not see (Dearborn Resources 2009) Introduces proper time as fourth dimension and uses circular diagrams for velocities in space and time. David Eckstein Epstein Explains Einstein (free ebook 2009) An explanation of Epstein's book

5D Space-Time-Matter consortium, formerly coördinated by the late Prof. Paul Wesson. Not so much identifiable as Euclidean relativity but proposals very similar to the ideas in it regarding the application of a fifth dimension, based on the Campbell-Magaard embedding theorem.
A quote from an article of Paul Wesson, In Defense of Campbell's Theorem as a Frame for New Physics (arXiv.org gr-qc/0507107, July 25th 2005) reflects one of the key elements in van Linden's article "The Universe as a Multi-Dimensional Fractal" :

"The implication of this for particles is clear: they should travel on null 5D geodesics. This idea has recently been taken up in the literature, and has a considerable future. It means that what we perceive as massive particles in 4D are akin to photons in 5D."

This site is maintained by Rob van Linden (rob@vlinden.com). Updated September 2024.